
High School
Student Workbook
PHYSICS DAY AT LAGOON
1998
Artist: Shannon Hawkins, Box Elder High School
STUDENT
TEACHER
HIGH SCHOOL
Amusement Park
Physics Glossary
Here are some physics concepts which you will
encounter today. Most of them should be familiar to you after the
exciting physics class you've been in this year.
ACCELERATION: Time rate of change of velocity (either speed or
direction)
of motion.
ACCELEROMETER: A device to measure acceleration.
AIR RESISTANCE: Force resisting motion of a body through air due
to the
frictional forces between the air and body.
AMPLITUDE: The maximum height of the wave above or below zero
level.
ANGULAR ACCELERATION: Time rate of change of angular
velocity.
ANGULAR VELOCITY: Time rate of change of angular position.
CENTRIPETAL FORCE: A force on an object pulling or pushing the
object
towards the center of its curved path.
CONSERVATION OF ENERGY: Basic tenet of physics stating that
energy
can neither be created nor destroyed in any process, though it may
change form.
CONSERVATION OF MOMENTUM: The total momentum of a system
is constant whenever the net external force on the system is
zero.
ELASTIC COLLISION: A collision in which kinetic energy is the
same
before and after the collision.
FORCE: A push or pull. The time rate of change (direction and
magnitude) of
momentum.
FREQUENCY: The number of waves that pass a particular point in
onesecond.
FRICTION: A retarding force that resists the motion of a
body.
G-FORCE: Ratio of the magnitude of acceleration on a body to the
acceleration
of gravity at sea level on Earth (g = 9.8 m/s2).
GRAVITY: Attractive force between two bodies, proportional to
their masses.
IMPULSE: Product of the magnitude of a force on a body times the
time over
which the force acts on the body.
INELASTIC COLLISION: A collision in which kinetic energy
decreases as
a result of the collision.
INERTIA: Tendency of a body to remain at rest or in uniform
motion in a
straight line.
Page 3
KINETIC ENERGY: The energy of a body associated with its
motion.
LONGITUDINAL WAVE: A wave that vibrates or oscillates in the
same
direction that thewave pattern is moving (example: sound wave).
MASS: The amount of material a body contains. A quantitative
measure of the
inertia of a body.
MOMENTUM: The product of mass times velocity.
NEWTON'S LAWS OF MOTION: Physical laws governing the motion of
bodies (at speed much less than the speed of light) expressed in
terms of
force, mass, and acceleration.
PERIOD: The amount of time for one complete wave oscillation to
pass a point
in space.
POTENTIAL ENERGY: Energy of a body associated with its
position.
POWER: Rate of work done per unit time.
SPEED: The magnitude of velocity.
TRANSVERSE WAVE: A wave in which the vibration or oscillation
is
perpendicular to the direction that the wave pattern is moving
(example:
stadium wave football cheer).
VELOCITY: The magnitude and direction of the time rate of
change of
position.
WAVELENGTH: The distance between successive crests or troughs
of a wave.
WEIGHT: A force proportional to the mass of a body.
Measurement of the
gravitational attraction of a body to the Earth.
WEIGHTLESSNESS: A condition under which a body feels no net
force
proportional to its mass.
WORK: Product of the magnitude of force on a body times the
distance through
which the force acts.
Useful conversion factors:
1 in = 2.54 cm 1 J = 2.78 x 10-7 kw hr = 9.5 x 10-4 BTU
1 km = 0.621 miles 1 W = 1J/s = 1.3 x 10-3 horsepower
1 liter = 0.264 gal 1 w = 1.3 x 10-3 horsepower
1 hr = 3600 sec 1 g = 9.8 m/s2 = 32 ft/s2
1 fortnight = 1.728 x 10
6 sec 1 N = 0.225
lb
1 m/s = 3.6 km/hr = 2.24 mi/hr 1 atm = 1 x 10
5 Pa = 14.7
lb/in2
1 Calorie = 1 kcal = 1000 cl =
4186J 1 kg/liter H
2O = 8.35 lb/gal H2O
Page 4
WOODEN ROLLER COASTER

1. Determine the angles of ascent and descent of the first
hill.
2. Identify at least three (3) sources of friction in this
ride.
3. Complete the diagram by putting in the proper labels. Label
the following, minimum potential energy, G; maximum potential energy, X;
maximum kinetic energy, K; minimum kinetic energy, M; weightless
sensation, W; heavy sensation, H.
4. What is the average speed from the beginning to the end of
the ride?
What is the average velocity?
Page 5
BUMPER CARS

1. What happens in a collision
to each car when:
a. one bumper car is not moving?
b. a rear-end collision takes place?
c. a head-on collision takes place?
d. bumper car hits a stationary object, i.e. the wall?
e. cars sideswipe each other?
2. What is the difference between two pieces of clay that
collide and two marbles
that collide? Do bumper cars behave more like clay or
marbles?
3. When will a driver:
a. feel the strongest jolt?
b. be thrown forward?
c. be thrown backward?
d. be accelerated?
Page 6
MUSIK EXPRESS
QUESTIONS
1. As you go over the high point you feel (heavier, lighter, no
change).
2. When loading each car, the (more massive, less massive) rider
should be seated on the outside. Why?
3. The more massive rider requires (a larger, a smaller, the
same) force to hold on the inside.
4. The centripetal acceleration on a large rider is (more than,
less than, the same) as on a small rider.
5. Explain how the ride would be different without the hills and
valleys.
6. Does the pitch of the music remain constant during the ride?
Explain.
Collect the following data for use with later
calculations:
1. Estimated radii for inside and outside riders: m
m
2. Height of high point: m
3. Time for one revolution at the maximum speed: s
4. Total number of revolutions in one ride:
5. Time for one ride: s
Page 7
FLYING ACES
MEASUREMENTS:
1. What is the time for a single revolution at top speed?
s/rev
2. What are the maximum and minimum radii of the planes and
their angles with respect to vertical?
Maximum: m ° Minimum:
m °
QUESTIONS
1. Planes move (toward, away from) the middle as they turn
faster.
2. What causes this to happen?
3. Although the center hub rotates at a constant rate, it does
not feel that way.
Why?
4. Diagram vector forces acting on the planes shown below.
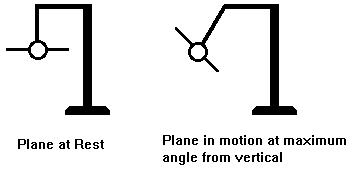
5. Calculate the speed of the planes in the two figures
above.
m/s m/s
Page 8
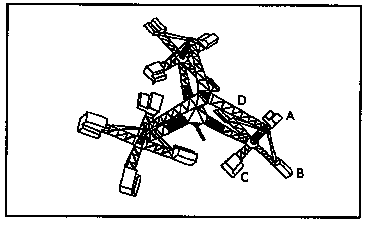
SCRAMBLER
|
Indicate the direction of rotation of
the ride cars about the main and secondary axes on the adjacent figure.
Sketch the path of the ride car.
How many rotations does the rider make about each axis?
Main ___________ Secondary __________
At which position (A, B, C, or D) was the speed (and force) of the ride
car a maximum?
At which position (A, B, C, or D) was the speed (and force) of the ride
car a minimum?
Calculate the total distance traveled by riding the Scrambler.
Graph the acceleration versus time for one main revolution of the
Scrambler. |

|
Page 9
Tilt-a-Whirl —
Chaos in Action!!!
|
When you hang a stone from a string, pull it aside, and let go the
stone swings back and forth in an almost exactly repeating way. These
repetitions are so regular that you can keep pretty good time with such a
simple toy. In fact, if you look inside a grandfather's clock you'll see
that the thing that "ticks" is a lot like a stone hanging f rom a
string.
The repetitive swinging of a stone hanging from a string is very
predictable. After you watch it for awhile it gets kind of boring. There
are many things in nature that repeat like a swinging stone. For
example, the sun comes up every day, the moon is full every month, the
temperature gets hot every summer, your heart beats about once every
second (at least, if you are sitting still and not watching a horror
movie). Those things, just like the swinging stone, are predictable.
|
|
On the other hand, there are lots of
other things which don't repeat in such a nice fashion and are very
unpredictable. Some examples are the gurgling ripples in a rushing
stream, the stretching and puffing of clouds blown by the wind, the
flickering of a candle's flame, and the faces of a pair of dice that come
up when you roll them.
Why do you suppose that some things are so regular and others so
unruly? Maybe some parts of nature are governed by rules and are tame
while other parts are run by luck and chance and are wild. Maybe.
But, hold on a second. If you make a gurgling stream slow down
enough the gurgles go away. And if you make the flame burn in just the
right way it becomes as smooth and regular as can be. Hmm.
But, the situation's more complicated still. The summer is hot
every year, all right, but never quite the same -- sometimes boiling,
sometimes okay. And if you time your heartbeat very carefully you'll find
it isn't regular either -- sometimes it's a little fast, sometimes a
little slow.
There are good reasons to believe that tame and wild behavior are
both governed by rules -- they are opposite sides of the same coin.
People are beginning to realize that some things that appear to be run by
chance and to be unpredictable (streams and flames and hearts are all
examples) actually march to the same kind of rules as the swinging stone.
We call such unruliness "chaos."
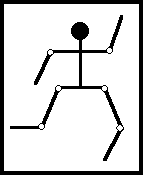
You may have seen a toy like the one pictured in the box. Its arms
are free to flail about, as are its legs. The whole body rotates around a
pivot in its chest. When you give the toy a good spin around its pivot,
the arms and legs go every which way without any seeming rhyme or reason.
If you give it a gentle spin, the arms and legs swing back and forth in a
regular, repetitive manner. In both cases, the arms and legs go where
rigid rules tell them to go. The wild, unpredictable behavior in this toy
isn't due to chance. It's chaos.
|
|
The Tilt-a-Whirl ride at Lagoon is
similar to this toy. Notice how unpredictable the ride feels. Pick a
spot on the ground. Every time you pass that spot jot down (if you can)
which way you're facing. Later, after you can think straight again, look
at your record. Does it seem repetitive or irregular? The irregularity
of the Tilt-a-Whirl is not due to some genie playing chance with you.
This ride obeys rigid rules. The unexpected whips and turns you just
experienced are chaos.
(So here's an interesting question for you to ponder when you have
nothing better to do: When you have an accident or you have a little good
luck or it rains when it isn't supposed to or you decide to eat a hot dog
instead of a piece of pizza, is that because of chance, or are you doing a
chaotic dance just like the toy? |
Page 10
SKY COASTER

1. Measure the half-period
(T/2) of motion for the first half-cycle, second half-cycle,
etc., until the ride comes to a stop. Does the period change
as the amplitude of the motion decreases?
2. Provide an explanation for the change in half-period T/2
measured in (1).
3. If there were no air resistance, would the period T be longer
or shorter?
Can you support your answer by observing the ride with
different numbers of riders?
4. From the period T for the smallest oscillations estimate the
height of the ride.
(Remember that T = 2¹(L/
g)1/2 where L is the length of the pendulum and
g = 9.8 m/s2 is the acceleration due to gravity.
5. Would the ride take longer on the surface of the moon? Why
or why not?
Page 11
CENTENNIAL
SCREAMER
|
Ride Facts :
Number of carriers: 20
Number of people per seat: 2
Length of ride: 1.30 minutes
Length of time between rides: 2.00 minutes
Radius: 6 to 8 m
Minimum rotational period: 4s
Maximum angle of tilt: 85°
|

|
1. Calculate the minimum speed necessary to stay in your seat at
the top of the ride.
2. Calculate the frequency of the ride at full speed.
3. Measure the angle each car makes with the vertical as the
wheel approaches full speed while still rotating horizontally. Is each
car uniformly the same angle, regardless of its position around the
wheel?
4. Calculate the circumference of the ride.
5. Determine the tangential speed of the ride.
Page 12
FERMI QUESTIONS
|
Enrico Fermi was one of this country's
greatest physicists. Among his accomplishments were the 1938 Nobel Prize
for nuclear and particle physics and the title "Father of the Atomic Age"
for his role in building the first nuclear reactor. He had a rare talent
as both a gifted theorist and experimentalist. One of his legacies is the
"Fermi Question," an insightful question requiring both an understanding
of physics principles and estimation skills.
The Fermi Questions given below require information gathered for this
workbook, estimation and some clever thinking. The additional questions
provide hints for one possible way to figure out the
answer. |

|
1. How many times do you have to
ride Colossus to pay for your Lagoon entrance ticket? Assume an electric
motor is 30% efficient, lifts a fully loaded Colossus roller coaster (7200
kg) up the first hill on Colossus.
a. Calculate the power necessary to raise the train.
b. If Utah Power and Light charges 10 cents per kilowatt hour,
determine the cost of the electricity used to power this ride for one
hour. Assume the ride to be operating at maximum capacity of a new train
every two minutes.
c. So, how many times do you have to ride Colossus?
2. Go check out the Skyscraper Ferris Wheel. This is one big,
bright ride! Is it bigger or brighter?
a. How much mechanical power is required to turn the ferris
wheel? Estimate the radius, mass and angular velocity to calculate the
power needed to form the ferris wheel. Assume the wheel turns 50% of the
time and that the electric motor is 30% efficient.
b. How much power is required to run the lights? They are 40 W.
bulbs.
c. Does it take more power to turn the ferris wheel or light
it?
Page 13
MEASUREMENT AND STATISTICS
1. Estimate the number of
people per hour that ride the Sky Tram. Count the number of riders
passing a certain point in one minute, then multiply by 60. Enter your
result as Trial 1 on the histogram below. Repeat your measurement. Find
two friends and "borrow" their results. Finish the histograms and
calculate the average number of riders per hour and the standard
deviation. The standard deviation tells you how well all the measurements
agree with each other.
| |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
1,600 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
1,500 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
1,400 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
1,300 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
Number |
1,200 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
1,100 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
of Riders |
1,000 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
900 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
per Hour |
800 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
700 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
600 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
500 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
400 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
300 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
200 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
100 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
|
|
|
|
1 |
|
2 |
|
3 |
|
4 |
|
5 |
|
6 |
|
|
| |
|
|
|
|
|
|
|
|
|
Trial |
|
|
|
|
|
|
Average 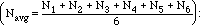
 :
:
2. Now measure the number of
riders passing your special point for 3 minutes.
Which gives the most precise result: a) the 3-minute measurement;
b) Trial 1;
or c) the average value from Part 1? Why?
3. Imagine you measure the length of the Sky Tram using three
devices:
1) a meter stick; 2) pacing the distance; and 3) a car odometer.
Which will give you the most prsecise value? Why?
Page 14
Physics Day at
Lagoon
Registration at
ENTRANCE
All Activities at DAVIS PAVILION
Page 15
Schedule of
Events
Time
Event Location
9:30 Lagoon Park opens
9:30-11:00 School & teacher registration Main Gate
9:30-11:00 Contest registration & safety Davis Pavilion
approval inspections
11:00 Curriculum Development entries due Davis Pavilion
11:00-1:30 Colossus' Colossal G-Forces Contest
2:00 Entry forms due Davis Pavilion
10:00-4:00 Physics Bowl Competition
10:15-10:45 Preliminary Qualification Round Davis Pavilion
11:00-12:30 Round of thirty-two Davis Pavilion
1:00-2:00 Round of sixteen Davis Pavilion
2:00-2:30 Quarter-final round Davis Pavilion
2:30-3:00 Semi-final round Davis Pavilion
3:00-3:30 Consolation round Davis Pavilion
3:30-4:00 Championship round Davis Pavilion
11:00-3:00 Physics Demonstration, Davis Pavilion
Lagoon: 2001 Ride Design, &
Physics Day Logo Design Contests
10:00-3:00 Student Workbook
3:30 Entry forms due Davis Pavilion
12:00-1:00 Faculty and staff complimentary lunch Gas Light
Terrace
3:30 All contest entry forms due at Davis Pavilion
registration desk
4:00 All rides close
4:15-4:45 Awards and closing ceremony Davis Pavilion
5:00 Park closes
••••••••••••
Win free
Day Passes and Season Passes to Lagoon
or
Space Posters!!!!
••••••••••••
All students who
turn in their workbook to the table at Davis Pavilion
by 3:30 have a chance to win fabulous prizes by random
drawing.
Workbooks must be turned
in by 3:30!!!
Page 16












